Jérémie Bettinelli
École polytechniqueLaboratoire d'informatique (LIX)
91128 Palaiseau Cedex
FRANCE
E-mail: firstname « . » lastname « at » normalesup « . » org
Office: 2023
Phone: (+33) (0)1 77 57 80 61
Jérémie BettinelliÉcole polytechniqueLaboratoire d'informatique (LIX) 91128 Palaiseau Cedex FRANCE E-mail: firstname « . » lastname « at » normalesup « . » org |
Désolé, pas de version française |  |
Stack triangulations |
back to map simulations |
You will find here simulations of stack triangulations. These maps are obtained from a map with two triangular faces by iteratively splitting a face into 3 new triangles. We consider two very different ways of chosing such a triangulation at random. The models presented on this page illustrate the results of Marie Albenque and Jean-François Marckert's paper entitled Some families of increasing planar maps.
Let us first look at what happens if we start from a map with two triangular faces and then, we iteratively split a face chosen uniformly at random into 3 new triangles.
| Iterative Stack 20k [jpg1 – jpg2] Iterative Stack 50k [jpg1 – jpg2] |
| Iterative stack triangulation with 20 000 faces and 50 000 faces |
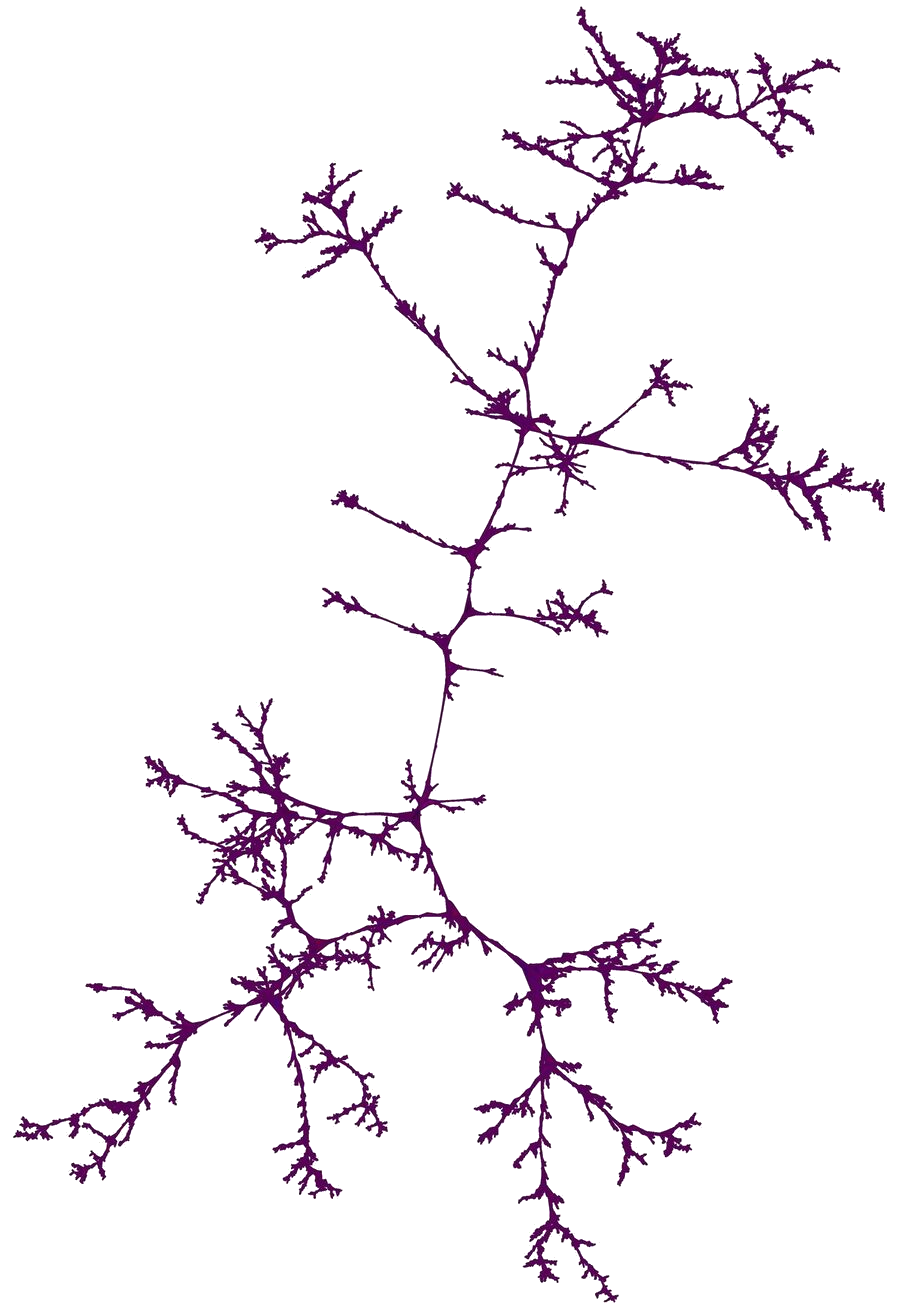
We now consider a stack triangulation taken uniformly at random among all stack triangulations with a given number of faces. It is shown in Some families of increasing planar maps that this object has the same limit as a uniform tree (compare with the simulation of a uniform random tree).
| Uniform Stack 20k [jpg1 – jpg2] Uniform Stack 50k [jpg1 – jpg2] |
| Uniform stack triangulation with 20 000 faces and 50 000 faces |